A rhombus might look complex with its tilted sides and unusual angles, but calculating its area is actually quite straightforward once you understand the underlying principles. It is crucial to know how to calculate an area when studying geometry. This article explores the rhombus, its defining characteristics, and the formulas used to determine its area, complete with step-by-step examples.
What is a Rhombus?
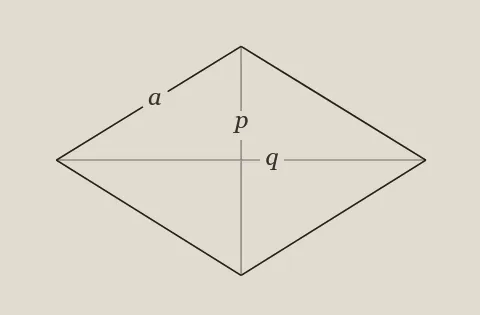
A rhombus is a type of quadrilateral where:
- All four sides are equal in length.
- Opposite angles are equal.
- Diagonals bisect each other at 90°.
- Diagonals divide the rhombus into four congruent right triangles.
While a rhombus shares similarities with a square (a special case where angles are 90°), it does not require right angles. This flexibility makes it a versatile shape in mathematics and real-world applications.
Formulas for Calculating the Area of a Rhombus
There are three primary methods to calculate the area of a rhombus, depending on the given information:
1. Using Diagonals (d₁ and d₂)
The most common formula leverages the lengths of the diagonals:
Area = d1 × d2 / 2
How it works: The intersecting diagonals create four right triangles. Each triangle has legs of d1/2 and d2/2. Summing the area of all four triangles gives the total area.
Example:
If diagonals measure 8 cm and 6 cm: Area = 8 × 6 / 2 = 24 cm
2. Using Base and Height
Similar to a parallelogram, the area can be calculated by multiplying the base (b) and height (h):
Area = b × h
Note: The height is the perpendicular distance between two parallel sides.
Example:
If the base is 5 cm and the height is 4 cm: Area = 5 × 4 = 20 cm
3. Using Trigonometry (Side Length and Angle)
If one interior angle (θ) is known, use the formula:
Area = b2 × sin(θ)
Here, b is the side length, and sin(θ) relates to the height (h = b × sin(θ)).
Example:
For a rhombus with side 10 cm and angle 30°: Area = 102 × sin(30°) = 100 × 0.5 = 50 cm
Why Do These Formulas Work?
- Diagonals Method: The diagonals split the rhombus into four congruent triangles. Doubling the area of one triangle (d1 × d2 / 4) accounts for all four.
- Base and Height: This mirrors the parallelogram formula, as a rhombus is a special case with equal sides.
- Trigonometric Approach: Derives from expressing height in terms of the side length and sine of an angle.
Common Mistakes to Avoid
When calculating rhombus area, be careful not to confuse the slanted side length with height. The height is always shorter than the side length unless the rhombus is actually a square. Also, when using the diagonal method, remember that diagonals must be measured from corner to corner, not along the edges.
Key Takeaways
- A rhombus has equal sides, perpendicular diagonals, and opposite angles.
- Area can be calculated using diagonals (d1 × d2 / 2), base-height (b × h), or trigonometry (b2 sin(θ)).
- Understanding these formulas empowers problem-solving in geometry and beyond.
Also Read: Calculating Total Seconds in a Year: A Detailed Explanation
Conclusion
In short, the rhombus, despite its appearance, is easy to understand and its area is simple to calculate. Knowing the different formulas, whether using diagonals, base and height, or trigonometry, gives you the tools to find the area of any rhombus.

